Workshop Statistics: Discovery with Data, Second Edition

Topic 21: Tests of Significance I: Proportions

Activity 21-1: Cola Discrimination (cont.)

(a) .333
(b) This value is a parameter since it's describing the underlying true probability, q
(c) The sample distribution is approximately normal with mean .333, and standard deviation .086.

(d) Yes, the sample size is large enough so that nq> 10 and n(1 - q) > 10.
(e)

probability: .0001 (z=3.88)

(f) Based on this probability, we would consider such a sample result very surprising if someone is just guessing. We would not consider it so surprising if we believed that the subject is not just guessing, but really does have some ability to discriminate among the sodas.
(g) Let q = true probability of correctly identifying the second brand
(h) H_o: q = .333 (they are just guessing)
(i) .333
(j) H_a: q > .333 (their actually probability is higher than .333)
(k) No, sampling variability.
(l) A: .333; B: .400; C: .500
(m) These proportions are statistics because they describe samples, not the population.
(n) yes
(o) .086
(p)
(q) .78
(r) .2177
(s) It is not very unlikely.
(t) test statistic: 1.94; p-value: .0262
(u) Yes, based on this p-value, we would reject the null hypothesis at the .05 significance level that Celia is just guessing because .0262 (the p-value) is less than .05.
(v) No, because the p-value is not less than .01.
(w) No, because her proportion is .333, which is equal to the hypothesized value. Since her sample proportion already wasn't better than what we'd expect if she's guessing, it will not provide evidence against that hypothesis.
(x) Neither Alicia nor Brenda showed sufficient evidence to convince us they were doing better than guessing. Celia, however, had a sample result that would happen in about 2% of samples if someone was just guessing. This is moderate evidence against the null hypothesis that she is just guessing. Instead we might be willing to conclude she has the ability to discriminate among the three sodas.
(y) 30 * .333 > 10 (actually, it equals 9.99, but this is only because we cannot use a decimal form of 1/3 without truncating the number of digits)
30 * (1 - .333) > 10

Activity 21-2: Baseball "Big Bang"

(a) H_o: q = .75 (A big bang occurs in 75% of Major League Baseball games)
(b) H_a: q < .75( A big bang occurs in less than 75% of Major League Baseball games)
(c)

<-----------------

------------------->
(d) .515
(e) Yes, this sample proportion is less that .75 and therefore consistent with Marilyn's (alternative) hypothesis.

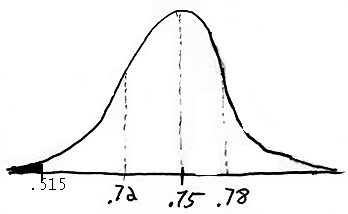
(f) z = -7.48; p-value < .0003
(g) Based on this p-value, the sample data provide very strong evidence to support Marilyn's contention that the proportion cited by the grandfather is too high to be the actual value because the p-value is much less than .001.
(h) H_o: q = .5 (Marilyn's claim)
H_a: q¹ .5 (Marilyn's claim is wrong)
(i) z = .44; p-value = .663
(j) Based on this p-value, the sample data provides no evidence to reject Marilyn's claim, since .663 > .1. We would fail to reject H₀, the sample data does not give us evidence to disprove Marilyn's conjecture. We'd get a sample result like this in about 66% of samples if q = .5. Doesn't seem very unusual.

Activity 21-3: Flat Tires

(a) The variable of interest in this activity is which tire people will pick at random, categorical.
(b) The parameter of interest q= the proportion of American drivers (or of students at your school) who pick the right front tire.
(c) .25
(d) H_o: q = .25 (people are equally likely to pick the right front tire)
(e) H_a: q > .25 (people choose the right front tire more often than they would if they were picking at random)
(f)-(g) Answers will vary from class to class.
[Check nq_o and n(1-q_o)]

Activity 21-4: Flat Tires (cont.)

(a) We would need to know the sample size in order to answer this question.
(b)-(c)

sample size	"right front"	p-hat	z statistic	p-value	alpha = .10?	alpha = .05?	alpha = .01?	alpha = .001?
50	15	.30	0.82	.207	no	no	no	no
100	30	.30	1.15	.124	no	no	no	no
150	45	.30	1.41	.079	yes	no	no	no
250	75	.30	1.83	.034	yes	yes	no	no
500	150	.30	2.58	.005	yes	yes	yes	no
1000	300	.30	3.65	.000	yes	yes	yes	yes

(d) Sample size plays a key role in determining whether a sample result of 30% is significantly greater than a hypothesized value of 25%. With a large sample size, as we see when n = 1000, a sample result of 30% is significantly greater than the hypothesized value. Eventually, for a big enough sample size, any result will be significantly greater than the hypothesized value!